Simple and Compounding Pendulums

Experiment for studying simple harmonic motion and the factors that affect the period of oscillation of pendulums. Fits on to the Free Vibrations Test Frame.
This product is part of a range that explores free vibrations in simple ‘one degree of freedom’ systems.
This product includes a rod and a circular plate to show the principles and use of simple harmonic motion theory in bifilar and trifilar pendulums. Two suspension plates fix to the top of the Test Frame to hold the bifilar and trifilar pendulums. Bifilar and trifilar, meaning of two (bi) or three (tri) threads or wires.
Students test the two pendulums to see how different factors, such as mass, support position or pendulum length affect the period of oscillation. The theory shows how to predict the period of oscillation of a given pendulum for comparison with actual results. The module includes an ‘example machine element’ in the form of a flywheel with spokes. This machine element fits on each pendulum, rotating around two different axes of rotation. It helps to show how you may use a pendulum to predict the moment of inertia of a part rather than use extensive calculation and measurements.
TecQuipment provide 3D CAD (Computer Aided Design) model files of the example machine element used in the experiments. The files are in several formats for use with the most popular 3D CAD software packages. This allows students to compare a software predicted moment of inertia against that found by experiment.
It introduces students to key scientific terms such as:
This product fits to the sturdy Test Frame (TM160) for study or demonstration.

Experiment for studying simple harmonic motion and the factors that affect the period of oscillation of pendulums. Fits on to the Free Vibrations Test Frame.
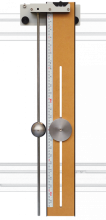
An experiment that illustrates how to calculate and find a compound center of percussion pendulums. Fits on to the Free Vibrations Test Frame.

Experiment that uses simple harmonic motion theory to demonstrate how to calculate the frequency of oscillation of simple mass-spring systems; demonstrates Hooke's law. Fits on to the Free Vibrations Test Frame.
